Physics Equations 01

Learning Physics 01

Back to Physics Mathematics Chemistry - - - Back to Home

I decided to learn Theoretical Physics after finding some video lectures from Stanford University taught by Leonard Susskind. They were fascinating but I could only follow the bare bones of what was being taught. I figured I needed to fill in the holes I had in Mathematics. As I delved deeper into trying to build a firm foundation on which to build I found out that I really did not know very much. I want to understand what this universe was made of and in so doing I would gain a knowledge of how that stuff interacted. At every turn I found more avenues that piqued my interest.

The Mathematics of the 17^th, 18^th, and 19^th Centuries reads like High Adventure exploring new vistas and yes, filled with intrigue. The scope of Set Theory, Geometry, and Algebra are truly mind bending. Concepts and tools developed from those times onward devoured my time .Eventually, I digested enough scraps of information and clarified my goal. I am where I am and this is how I will get to where I will be.

I want to see the Equations of Physics as stuff interacting with stuff. I want to see the Form and Motion. So, I need to know the language. What follows is a fairly narrow track of pronunciation and definitions. I have realized that a definition in a living language does not stand in isolation. It is the inter connectivity of words that create concepts that resolve to comprehension.

Is this anything?

Physics Mathematics Chemistry
jimw@umich.edu Physics Equations 01.pdf

Learning Physics 01
Is this anything?
Are Stuff and Interaction two things? I don't know.
Calculus, Algebra, Geometry, Trigonometry: What requisite parts?
Straddling Mathematics and Physics
Physics Equations are Models
It gets Bumpy from Here
Enter the Limit
Coordinate Systems
You are Here
Well, Just a few Steps
Coordinate Systems: We have 1; Lets go for 2
A Closer look at Vectors
Vector Multiplication comes in Two Forms
A Coordinate System in 3-Space to Finish Vector Multiplication
A Coordinate System in 3-Space...
...to Finish Vector Multiplication
Coordinate Systems: A Last Word for now
2-D Space
3-D Space

OK, Physics
First Experiment
Second Experiment
Third Experiment
Forth Experiment
A Handful of Fundamentals
Galilean Transformation
What does Physics measure?
Length[L], Time[T], and Mass[M] are Fundamental for now
Perception and Prediction: Part I
A Closer Look at Newton's Laws of Motion
This Should Really Be A Footnote: E1
This Should Really Be A Footnote: E2
A Comment On Refinement
IOTTMCO Discoveries Explained
Acceleration due to Gravity, Weight, Normal Force, and Friction
This is not a Tricky Question: Throwing Stones
Motion and Gravity
Einstein's Field Equations are a Piece of Cake
Paths not Taken

Physics is an experimental science. Measured quantities are known in Physics. Physics endeavours to express these quantities in their fundamental form. An experiment detects an interaction between the apparatus and the thing being measured. An experiment gives reproducible results anywhere it is performed in the Universe. These constraints on observations allow the Physics Equation to model the stuff and interactions.

Are Stuff and Interaction two things? I don't know.

Experiments give rise to data which Physics Equations model in a fundamental form. Newton is credited with this Law: "For every action, there is an equal and opposite reaction.". The short rewrite F₁₂ = - F₁₂ fits into the larger schema of Physics. When thinking or speaking Physics Equations it is helpful to pronounce them correctly to facilitate communication. Many branches of Mathematics provide the Relationships of the Observable's in Physics.

Mathematics is a rich science of diverse content. Discovering what content and depth to comprehend Physics is an intrepid expedition. Encountering Sirens can be anticipated, but recognizing them is another matter. Fortunately, no knowledge is wasted and a dalliance with a Siren is often rewarding. The Observer affects what is being Observed. The needs of Physics might be less than what Mathematics has to offer. A Drunken Walk might be the path to comprehending Physics Equations.

To bypass the Mathematical Drunken Walk go directly to...OK, Physics

Calculus, Algebra, Geometry, Trigonometry: What requisite parts?

A derivative is a specific case for a Limit. A Limit performs an action on a function. A function defines a relationship among variables. A variable is a measured quantity. Functions come in Families. Mathematics defines a function and gives its meaning to those who can read mathematics. Mathematics relates one family member to another. A function and its derivative are members of a family.

I heard Richard Feynman say, "Mathematics is more than a Language. It is Language and Reason." Between each symbol of a function and between each function of a family is a logical structure. You can comprehend Mathematics by understanding the Symbols and the Logic. You need both just for mathematics. Physics Equations need Mathematics to model the Stuff and Interactions. The values of the stuff and interactions are measured or calculated quantities in Physics. You can comprehend Physics by understanding the Symbols and the Logic.

Position, velocity, and acceleration is a Family. Mass, momentum, and force is a Family. Mathematics relates these two families but that is a path not taken at this time. The path of position, velocity, and acceleration illustrates a derivative family of three members in the intersection of the Mathematical and Physical Realms.

₁
x_f = x_i + v_xit + --- a_xt²
²

The final position

equal the initial position plus the initial velocity plus one-half the acceleration multiplied by time squared.

Straddling Mathematics and Physics

A function operates on a variable that results in another variable that is dependent on the function as a definition and the initial variable as its input. The range of the input variable is restricted by what is or can be measured and the logic of the function when it processes that input. The output exists within the domain of the functional operation. The function f of x equals y is written y = f(x). The labels f, x, and y are conceptually arbitrary but there are centuries of tradition that dictate how one talks these things in polite company.

In the foundations of Calculus is the Limit operator. There is a special case of the Limit which is defined as the Derivative operator. I hear the Siren's Song just thinking about the Limit. Physics Equations need the Limit, but the vast scope and awesome power of the Limit makes it difficult to stay my course to a single path when I glimpse the vistas of this Magnificent Realm. I fear and freely admit I will not do justice to the Limit as an Artisan may, nor as a mechanic who crafts their own tools. I resign myself to planting a seed acquired from this Mathematical land.

Physics Equations are Models

The models are specific definitions of positions, motions, and forces. They represent the properties and relationships of stuff and interactions. When a function operates on an input it produces and output. Supplying a different input renders a different output.

y₁ = f(x₁) and y₂ = f(x₂)

In this specific case where x₁ changes to x₂ results in y₁ changing to y₂. The change in y as the result of the change in x is the average rate of change of the function f(x).

y₂ - y₁ Δy₂₁
-------- = ----
x₂ - x₁ Δx₂₁

is the average rate of change of the function between two specific points. Now, let x be any two arbitrary values in the range of f(x) that are very close to each other. Choose the initial and final values of x even closer together than x₁ and x₂ are.

y_f - y_i Δy
-------- = ----
x_f - x_i Δx

This re-write is more general but it remains the average rate of change of the function albeit now between two arbitrary points. Proving that one can select an input value arbitrarily close to another input value that produces a valid output value will also produce a valid output value is "really cool". A mathematician would say simple and elegant, but this to is a path not taken at this time.

It gets Bumpy from Here

Notice that x_f - x_i = Δx and can be written x_f = x_i + Δx where both x_f and x_i are still within the range of f(x). Also, y_f - y_i = Δy and can be written y_f = y_i + Δy where both y_f and y_i are still within the domain of f(x). In this neighbourhood of x_f, x_i, x₂, x₁, and y_f, y_i, y₂, y₁ let x, y, and y = f(x) exist and be continuous. The concepts of "neighbourhood" and "continuous" are a result of "a path not taken". So y = f(x) can also be written as y = f(x + Δx) which also exists and is continuous. The average rate of change of the function can be written as

Δy f(x + Δx) - f(x)
---- = ------------------
Δx Δx

Replacing the label f() with the function, say this one

                           ₁
x_f = x_i + v_xit + --- a_xt²
                           ²
where x_f is a label for function of position over a range of time
Since x_f is just a Label it can be replaced by P(t) which means the same thing. It is easier for me to see that position is a function of time by writing P(t). Observable Quantities of Time come in and Observable Quantities of Length go out thus x = P(t).
x_i is the starting position measured in Length
v_xi is the starting velocity measured in Length per Time
and a_x is the acceleration measured in Length per Timed squared

Take note that x_i, v_xi, and a_x are constant values in this expression. Also the subscript _x in v_xi and a_x indicate these vectors are parallel to the x-axis.
The function maps Time onto Length and the symbols are identified.
                                     ₁
x = P(t) = x_i + v_xit + --- a_xt²
                                     ²

Substituting the Physics Equation P(t) into the average rate of change definition give rise to symbols that can be simplified using Algebra or Trigonometry.

Δx P(t + Δt) - P(t)
---- = -------------------
Δt Δt

                                                ₁                                      ₁
Δx         (x_i + v_xi(t + Δt) + --- a_x(t + Δt)²) - (x_i + v_xit + --- a_xt²)
                                                ²                                                ²
---- = ----------------------------------------------------------------
Δt                                                         Δt

                                                 ₁                                                               ₁
Δx           (x_i + v_xi(t + Δt) + --- a_x(t² + 2tΔt + Δt²)) - (x_i + v_xit + --- a_xt²)
                                                 ²                                                                ²
---- = -----------------------------------------------------------------------
Δt                                                           Δt

                                                  ₁                              ₁                                        ₁
Δx          (x_i + v_xit + v_xiΔt + --- a_xt² + a_xtΔt + --- a_xΔt²) - (x_i + v_xit + --- a_xt²)
                                                  ²                              ²                                        ²
---- = --------------------------------------------------------------------------
Δt                                                                    Δt

                                               ₁
Δx             v_xiΔt + a_xtΔt + --- a_xΔt²
                                               ²
---- = -------------------------------
Δt                               Δt

Note that the function P(t) relates position to time. This function relates the average rate of change of position to time.

Enter the Limit

The function that defines the instantaneous rate of change at every value of x requires that Δx = 0. Since division by zero is undefined the Limit operator is used to define this derivative as Δx approaches zero.

dy     _lim          f(x + Δx) - f(x)
---- =           -------------------
dx     ^{Δx -> 0}                Δx

dx     _lim           v_xiΔt + a_xtΔt + ½a_xΔt²
---- =           -----------------------------
dt     ^{Δx -> 0}                        Δt

Divide by Δt before it reaches 0

dx     _lim
---- =             v_xi + a_xt + ½a_xΔt
dt     ^{Δx -> 0}

Take Δt to 0

dx
---- = v_xi + a_xt = P′(t)
dt

A Derivative operating on a function results in a function defining the mechanism of change of the first function.

P(t) = x_i + v_xit + ½a_xt²
P′(t) = v_xi + a_xt
P′′(t) = a_x

The "derivative of f" has many names: "d dx of f", "d f", "f prime", "dy dx" "y prime", "y dot", and some other name mangling to designate the second, third, fourth derivative, and so on. A sampling of such notation is as follows

_dy _df _d
f ′(t) = y′ = --- = --- = --- = Df(x) = D_xf(x)
^dx ^dx ^dx

The derivative operator sheds light on the deeper structure of a function. The structure is there even when I don't know about it. Say the initial function was constructed from the observation of an apple falling from a tree or the swing of a pendulum. Or a thrown stone. Or the flight of a canon ball. Or the path of the Moon around the Earth. Or the orbits of the planets around the Sun. Truth be told, with careful measurement and an understand of what the symbols mean the Family of functions hold together the observations from apple to planets.

Coordinate Systems

Physics is an experimental science. The numerical measurements produce vast amounts of data which need to be organized so patterns can be discerned. Graphing the data is a powerful method to illuminate patterns. Each data point on a graph is a group of two or more measured quantities. Each point is a coordinate of the graph. If no data is plotted on a graph, the graph still has an axis or two.

Starting with a position one is confronted with the concept of something being "Over There". Beginning with a position and nothing came before this position I can go "Over There" I can come back to the original position. Being lazy and a poor typist I call the original position the "origin". In fact I can be at the the origin with out even having to go "Over There" right from the begriming. Recall the concepts of "neighbourhood" and "continuous" are a result of "a path not taken". They apply here. Two result are equally valid and both illustrate the meaning of "between".

There is a direction that starts from the origin that goes to "Over There" and beyond forever such that "Over There" is between the origin and forever. This mathematical structure is called a ray. There is over two thousand years of properties and implications concerning the ray and forever. Case in point, forever leads to the concept of infinity, ∞, which helped push the mathematician Georg Cantor over the edge. This alluring path will be a path not taken.

Well, Just a few Steps

The structure at hand is a directed ray from zero to infinity, specifically from zero to positive infinity. By putting a little hat^ on the variable e the shorthand, ê = (0, +∞) is read as "the vector e has an inclusive range of zero to positive infinity". So a vector has a length and a direction even when the length is zero. The length of a vector is called the magnitude of a vector. A magnitude without a direction is called a scalar. The operators addition, subtraction, multiplication, and division are defined for the scalar on a ray and illuminate another mathematical structure called a line. It was discovered that there can be a negative "Over There" such that 0 is between it and a positive "Over There". The range of a line is found to be from -∞ to +∞. The Line is a 1-Dimensional Space and the ray is a special case of a Line called a Half-Line. Three operations on vectors that are consistent in this 1-D Space are addition, subtraction, and multiplication by a scalar. "Which came first, the vector or the scalar"? Go wander around in Set Theory and Number Theory. Be sure to bring Bread Crumbs and 50 feet of rope.

Coordinate Systems: We have 1; Lets go for 2

Each position on a line has the same properties of every other position on the line. Observation of a line is an interaction with the line manifest as a coordinate system.

Recall that I have no answer to the question "Are Stuff and Interaction two things?" though I might pursue that line of inquiry at a later time. I am content with a 1-Dimensional Coordinate System such as it is.

A 2-dimensional system can be constructed in several mathematical ways maintaining the properties of scalars, vectors, rays, lines, and operators. Use a line (-∞, +∞) which is also a Set and pick a position to be the origin. Let the origin be zero, O = 0. Label this set X with elements from -∞ to +∞ where the element x = O_x = 0 can be written

X = {-∞ < x < +∞} |O_x ∈ X : x = 0.
Duplicate this set and label it,
Y = {-∞ < y < +∞} |O_y ∈ Y : y = 0.

Construct the union of X and Y, where the intersection of X and Y is uniquely defined by the ordered pair (x, y) as the origin where x = y = 0 for all ordered pairs,
(X ∪ Y) ∩ {O_x = O_y= 0} ≡ (0, 0) ∀ (x, y)

The 2-Dimensional space is a 2-D vector since X and Y are both 1-D vectors defining the ordered pair which technically is (xê_x, yê_y) but the "e hat" vector notation generally is dropped. The variables x and y in the ordered pairs are scalars multiplied by what are called the basis vectors ê_x and ê_y of the Cartesian Plane. The distance to any point on the plane from the Origin is given by the magnitude of the vector with its Tail at the Origin and its Head at the Coordinate (x, y). From the Pythagorean Theorem

In any right triangle, the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares whose sides are the two legs (the two sides that meet at a right angle)

where x and y are the legs and (0, 0) to (x, y) is the hypotenuse, call it s, s = √x² + y². Writing the relationship as s² = x² + y² looks better on the page and means the same thing. If s = 1,

then x² + y² = 1 defines a set of coordinates called the unit circle with center at (0, 0). The nice thing about 2-space is 2-D objects can exist. A vector having a magnitude of 1 with its tail at (0, 0) has its head on the circumference of the circle and is called the radius, r, of the circle. With the tail of vector r remaining at (0, 0) the head can start at (1, 0) and rotate counter-clockwise through (0, 1), (-1, 0), (-1, -1), and back to the initial orientation (0, 1). From the Pythagorean Theorem the length of the circumference is c = 2πr. So rotating an object through an angle of 360°, also written as ∠ 2π radians, returns an object to the initial orientation.

A Closer look at Vectors

Consider the set X which has a basis vector of length one and scalar values from -∞ to +∞, X = { ê_x ∧ -∞ < x < +∞}. For all scalars a, b, c, and d in X the following operators are defined

a + b = b + a = c
a × b = b × a = ab = ba = Σ₁^b a_i = Σ₁^a b_i = d

"multiplication is addition done many times for integers, the sigma, Σ, is a symbol for summation
Σ₁^b a_i = a₁ + ... + a_b, where b ≠ 0 and Σ₁^b a_i = 0 where b = 0"

a × 0 = 0 × a = 0
a × 1 = 1 × a = a

b × (-1) = -b
a - b = a + ((-1) ×b) ≠ b - a = b + ((-1) ×a), ∀ a ∧ b, "for all a and b"
"subtraction is addition of a negative number and is non-commutative"

d / a = b, where a ≠ 0 and d / a = 0, where a ≠ 0 and d = 0
"d/a can be an integer, fraction, rational, irrational, computable, 'more numbers, than are dreamt of in your philosophy', and numbers named due to their virtue fundamental to the universe such as π"

"the scope of multiplication as defined by the Σ operator is actually broader due to the ramifications of division and the basic concept remains intact"

Vectors in X = { ê_x ∧ -∞ < x < +∞} point in a direction and have a length. The direction of the basis vector, ê_x, is that direction. The length of ê_x is the scalar value 1.

1 × ê_x = ê_x
a × ê_x = aê_x
aê_x + bê_x = (a + b)ê_x = cê_x, where a + b = c

"graphically the head of a is placed at the tail of b and the length of c is from the tail of a to the head of b"

The vectors a, b, and c are all parallel to each other and the magnitude of a vector exists in the range of (0, +∞).

(-1) × ê_x = -ê_x

means that -ê_x is anti parallel to ê_x. If ê_x points in that direction, then -ê_x points in the other direction. To be consistent with the mountain of mathematics climbed so far multiplication of a vector by -1 rotates the vector through an angle of 180°, also written as ∠π radians.

aê_x - bê_x = (a - b)ê_x = cê_x, where a - b = c

"graphically the head of a is placed at the tail of b and the length of c is from the tail of a to the head of b"

Think of rotates through an angle in a 1-space as quantum mechanical states where ê_x points ∠ 0 and -ê_x points ∠ π with nothing between.

Vector Multiplication comes in Two Forms

The Pythagorean Theorem, "In any right triangle, the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares whose sides are the two legs (the two sides that meet at a right angle)" defines the relationships of the component magnitudes of a right triangle in 2-D space.

hypotenuse_xy² = leg_x² + leg_y²

In 1-D space leg_y does not exist and the relationship reduces to hypotenuse_x² = leg_x² This relationship gives rise to the useful definition of Absolute Value

|x| ≡ √x²

Interestingly, |hypotenuse| = |leg_x| = |h| = |xê_x| for a 1-D triangle for xê_x directed ∠ 0, parallel to ê_x, and xê_x directed ∠ π, anti parallel to ê_x. By considering all the mathematical properties, spaces, structures, and operations discovered thus far, it is reasoned that ratio

leg_x, the leg adjacent to ∠ 0 or ∠ π
-----------------------------------------
hypotenuse_x

is proportional to ∠ 0 or ∠ π in a significant and fundamental manner.

x
--- ∝ ∠ θ
h

", where θ = 0 and π in 1-space and θ = (0, +∞) in 2-space"

The proportionality function is the cosine

x
--- = cos θ
h

This is the bare minimum to define one form of vector multiplication, called the dot product.

a ⋅ b ≡ |a| |b| cos θ

In 2-space, a plane, vectors a and b can have an angular separation of any arbitrary value of θ. Recall that a rotation of ∠ 2π returns an object to its initial orientation, not surprisingly the range of the cosine function exhibits the same nature. Reducing the domain θ from (-∞, +∞) to 0 ≤ θ ≤ 2π maintains the full set of the range -1 ≤ cos θ ≤ 1. Cosine is a periodic function having a period of 2π.

No matter what the angle between a and b is, b can be written as the sum of two vectors that are perpendicular and parallel to the vector a

b = b_⊥ê_a⊥ + b_¶ê_a¶

"the parallel part, b_¶ê_a¶ is called the projection of b onto a and equals"

|b| cos θ

"in the definition of the Dot Product"

The Dot Product can be calculated from ordered pairs in a Cartesian Plane. Since each (x, y) coordinate is a vector with its Tail at the Origin and the Head at the point (x, y) where x is the projection onto the x-axis and y is a projection onto the y-axis.

For any two points (a_x, a_y) and (b_x, b_y)
a ⋅ b = a_xb_x + a_yb_y

This pattern of the Dot Product extends to 3 and higher dimensional spaces.

a ⋅ b = (a_x, a_y, a_z) ⋅ (b_x, b_y, b_z)
a ⋅ b = a_xb_x + a_yb_y + a_zb_z

Vectors are used extensively in Physics such as Work which is defined as the scalar product of force and displacement.

A Coordinate System in 3-Space to Finish Vector Multiplication

1-D Space	X = { ê_x ∧ -∞ < x < +∞} \|O_x ∈ X : x = 0
2-D Space	X = { ê_x ∧ -∞ < x < +∞} \|O_x ∈ X : x = 0 Y = { ê_y ∧ -∞ < y < +∞} \|O_y ∈ Y : y = 0 (X ∪ Y) ∩ {O_x = O_y= 0} ≡ (0, 0) ∀ (x, y)
3-D Space	X = { ê_x ∧ -∞ < x < +∞} \|O_x ∈ X : x = 0 Y = { ê_y ∧ -∞ < y < +∞} \|O_y ∈ Y : y = 0 Z = { ê_z ∧ -∞ < z < +∞} \|O_z ∈ Z : z = 0 (X ∪ Y∪ Z) ∩ {O_x = O_y = O_z= 0} ≡ (0, 0, 0) ∀ (x, y, z)

There is probably a more compact way to write three orthogonal sets of infinite numbered vector spaces but this verbose way maintains the visibility of the base properties. The unit vectors ê_x, ê_y, and ê_z orient the x-axis, y-axis, and z-axis. The coordinated of the unit vectors are

ê_x = (1, 0, 0)
ê_y = (0, 1, 0)
ê_z = (0, 0, 1)

Using the Dot Product show the 3 axes are perpendicular to each other

ê_x ⋅ ê_y ⋅ ê_z = (1 × 0 × 0) + (0 × 1 × 0) + (0 × 0 × 1) = 0

ê_x ⋅ ê_y= 0
ê_x⋅ ê_z = 0
ê_y ⋅ ê_z = 0
ê_x ⋅ ê_y ⋅ ê_z = 0

But, why is the x-axis positive to the right and negative to the left with the y-axis positive in the up direction and negative directed down having the z-axis positive coming out of the page and negative going into the page?

The result of Vector Multiplication by the Dot Product is a scalar. The Dot Product addresses parallel components of two vectors. The other form of Vector Multiplication is called the Cross Product which resolves to a vector. The Cross Product addresses perpendicular components of two vectors. Starting with the Pythagorean Theorem

hypotenuse_xy² = leg_x² + leg_y²

The leg of interest is

leg_y, the leg opposite to ∠ θ
----------------------------------
hypotenuse_xy

The significant and fundamental proportionality

y
--- ∝ ∠ θ
h

is defined as the periodic function called sine

y
--- = sin θ
h

The Cross Product

a × b ≡ |a| |b| sin θ

Results in a vector pointing in the right direction. To ensure the direction is correct there are two properties of the Cross operator that need to be addresses, right? The vector product is not commutative

a × b ≠ b × a

and the measured angle progresses from the first listed vector to the second listed vector. The left hand side of the equation measures θ from a to b and the right hand side measures θ from b to a, right?

"Truth be told,"
a × b = - b × a

To find the right direction of the resulting vector, take your right hand and wrap your fingers from the first vector to the second vector. Your thumb points in the fight direction. This technique is called, "The Right Hand Rule".

A Coordinate System in 3-Space...

The basis vectors ê_x, ê_y, and ê_z all have a positive magnitude and are perpendicular to each other. The most ergonomic way to draw a line on a page to represent the x-axis and possessing the foreknowledge that "The Right Hand Rule" will be applied to determine the directions of the other two axes is to draw a horizontal line with positive going to the right. This leads to positive y values traveling up the page and positive z values coming out of the page.

...to Finish Vector Multiplication

Calculating the vector product with basis vectors looks algebraically daunting, only because of the bookkeeping. It is worth walking through the operation to build some vector intuition which is very useful in Physics.

a × b = (a_xê_x + a_yê_y + a_zê_z) × (b_xê_x + b_yê_y + b_zê_z)
= a_xb_x(ê_x × ê_x) + a_xb_y(ê_x × ê_y) + a_xb_z(ê_x × ê_z)
+ a_yb_x(ê_y × ê_x) + a_yb_y(ê_y × ê_y) + a_yb_z(ê_y × ê_z)
+ a_zb_x(ê_z × ê_x) + a_zb_y(ê_z × ê_y) + a_zb_z(ê_z × ê_z)

The following list of properties are apparent from the concepts described thus far

a × (ab) = (aa) × b = a(a × b)
a × (b + c) = a × b + a × c
a × b = - b × a
a × a = 0

ê_x × ê_x = ê_y × ê_y = ê_z × ê_z = 0

ê_x × ê_y = −ê_y × ê_x = ê_z
ê_x × ê_z = −ê_z × ê_x = −ê_y
ê_y × ê_z = −ê_z × ê_y = ê_x

after some concentration

a × b = (a_yb_z − a_zb_y)ê_x + (a_zb_x − a_xb_z)ê_y + (a_xb_y − a_yb_x)ê_z

but this can be written as a determinant that is easy to remember and easy to use. The Determinant comes from Linear Algebra. To set up a determinant and calculate a × b Right Hand System

| ê_x ê_y ê_z |

a × b = det a_x a_y a_z

b_x b_y b_z

Multiply diagonal entries together going uphill from right to left for adding

+ (b_za_y)ê_x + (b_ya_x)ê_z + (b_xa_z)ê_y

Multiply diagonal entries together going uphill from left to right for subtracting

- (b_xa_y)ê_z - (b_ya_z)ê_x - (b_za_x)ê_y

Collect the terms

a × b = (b_za_y − b_ya_z)ê_x + (b_xa_z− b_za_x)ê_y + (b_ya_x− b_xa_y)ê_z

Which is the same as the standard algebraic result

a × b = (a_yb_z − a_zb_y)ê_x + (a_zb_x − a_xb_z)ê_y + (a_xb_y − a_yb_x)ê_z

but many fewer steps.

Coordinate Systems: A Last Word for now

Space has properties. A lot of Physics fits into this 3-D Euclidian space. As the need arises other properties of space will be explored. A Coordinate Systems is a mapping onto a space so the tools of mathematics can be used. The Laws of Physics do not depend on the Coordinate System used to express them.

2-D Space

Coordinates are operational functions the relate every point in space to the Origin of a Coordinate System. For a point, P, in a plane Cartesian coordinates are (x(P), y(P)) and Polar coordinates are (r(P), θ(P)).The functions are defined

x(P) ≡ the distance from the y-axis parallel to the x-axis
y(P) ≡ the distance from the x-axis parallel to the y-axis
r(P) ≡ the distance, radius, from the Origin, called the Pole
θ(P) ≡ the counter-clockwise angle, azimuth, from a fixed ray,
called the Polar Axis

and the symbol (P) are dropped from the notation. The placement of the Origin for Cartesian coordinates and Polar coordinates is completely arbitrary as is the orientation of the polar axis. The choice of coordinates is a matter of convenience.

3-D Space

Three convenient coordinate systems used in Physics are

Cartesian (x, y, z)

x ≡ the distance from the y-axis parallel to the x-axis
y ≡ the distance from the x-axis parallel to the y-axis
z ≡ the distance from and perpendicular to the x-y plane

Cylindrical (ρ, φ, z)

ρ ≡ the distance from the Pole and perpendicular the z-axis
φ ≡ the angle from the Polar Axis about the z-axis

"Polar (r, θ) = Cylindrical (ρ, φ, z) where r = ρ, θ = φ, z = 0"

z ≡ the distance from the Pole and parallel to the z-axis

Spherical (r, θ, φ)

r ≡ the distance from the Pole
θ ≡ the angle from |(Polar Axis)×∠φ|

"that is to say, θ ≡ the angle from the perpendicular projection of the reference plane resulting from the Polar Axis and the angle φ"

φ ≡ the angle from the Polar Axis

with the choice of which to use as dictated by simplicity and clarity of the Physical Processes. When transforming between these coordinate systems it is convenient to set Pole = Origin and Polar Axis = x-axis ≥ 0.

OK, Physics

A body in motion tends to stay in motion and a body at rest tends to stay at rest unless acted on by an external force. Centuries of experimentation to prove or disprove this statement led to the conclusion that the assertion is correct. This observation has stood the test of time and its implications have grown deep roots on Physics.

First Experiment

Imagine sitting in an airplane flying through calm sky at a velocity of 600 miles per hour. Place a coin on the tray-table, then trace its circumference with a pen. lift then coin and drop it several times. It falls straight down and hits the target within the experimental error of the setup.

Second Experiment

Now take the spring out of the pen and an ice cube from the complimentary beverage provided by the airline. Place one end of the spring against the lip of the tray-table. Place the ice cube on the table and at the free end of the spring. Move the ice cube toward the lip of the table to compress the spring. Release the ice cube. In less than half-a-blink-of-an-eye, a very short time indeed, the ice cube is moving across the table.

Repeating this experiment several times and at various angles on the tray-table:

side to side being left to right and right to left, front to back, back to front, corner to far corner, ...

and measuring the speed of the ice cube anywhere along its path just after a "blink" from release to just before colliding with the lip of the table

finds the path of the ice cube to be straight and the speed to be constant within the experimental error of the setup. It is also determined that lighter ice cubes move faster than heavier ice cubes.

Third Experiment

Since the pen is already in pieces place the ink cartridge flat in the table. Balance a rule across the cartage so that it is horizontal and the distance markings are visible. Select two ice cubes such that when each is placed on the rule equidistant from the cartridge the rule remains horizontal. The two ice cubes will have the same weight within the experimental error of the setup.

Forth Experiment

It is possible to set up the ice cube experiment and place the second cube on the table and predict whether or not there will be a collision between the moving and stationary ice cubes. The prediction is not Clairvoyance it is Physics.

Set up the second ice cube experiment such that a collision occurs each time it is repeated and the two cubes remain in contact after the collision. Measuring the velocity of the moving cube before the collision with the stationary cube and then measuring the velocity of the two cubes moving together reveals that the velocity decrease by a factor of two within the experimental error of the setup. Repeating the experiment with the two ice cubes already in contact with each other results in a constant velocity that is equal to the final velocity recorded for the collision experiment.

Image the airplane will be landing soon and being an honourable individual you clean up the mess made on the tray-table. After the airplane lands you await your ride sitting at a restaurant table moving at 0 miles per hour and order a beverage. Repeating the experiments done on the airplane render the same results.

A Handful of Fundamentals

Though the two sets of experiments were done in different environments, the airplane (A) traveling at a velocity of 600 miles per hour and the restaurant (R) traveling at 0 miles per hour, they share important similarities. In both A and R each "tick" of time is the same everywhere. In both A and R all points have the same properties and are uniform in all directions. What, at first glance, looks like the "difference" is actually a similarity. Both A and R are moving with a constant velocity.

The set of "ticks" and "points" moving with constant velocity is called an Inertial Frame.

When a Coordinate System is mapped onto an Inertial Frame it is called a Frame of Reference.

Physics today rests on a foundation of philosophy, history, mathematics, and experimentation. The final arbiter of the Validity of Physics is Experimental Reproducibility. Physics may not be the Truth (Capital "T" Truth) but it points in the direction of what is true (Small "t" truth). Our understanding of the "Laws of Nature" are Physics Equations and subject to refinement. Some fundamental concept may very well be Truly Fundamental while other are truly fundamental in utility and guidance.

Safer Physics ≡ retain a "so far so good" attitude and an eye toward Critical Thinking

The prospect of determining the laws that govern the physical world is based on a centuries old concept.

Principle of Relativity ≡ Physics Equations describing the Laws of Nature must have the same form for any Frame of Reference.

This extremely broad definition requires that all the special cases that are derived from it are consistent with each other.

The motion of the airplane (A) can not be detected by an observe in the airplane because the Coordinate System use to make observation is moving with the Inertial Frame_A. The same holds true in the restaurant (R) even though Frame_R is is moving about 1000 miles per hour relative to the Sun and stars. The coins fall straight down and the ice cubes move in straight lines in both Frame_A and Frame_R. The dropped coin does not change position along the airplane axis from tail to nose(X_A) for the Frame_A observer. However Observer_R sees the entire Frame_A displaced along the Flight Path(X_R).

In the restaurant (R), Observer_R tracks objects with (x_R, y_R, z_R, t_R) and records an airplane(A) flying with a velocity_{x_R}. Observer_A, aboard the airplane(A), tracks objects with (x_A, y_A, z_A, t_A). Both Observers Agree:

Each determines themselves to be at rest and the other is moving
Lengths are the same for each
Time is the same for each

Observer_R derives the following coordinate transformation:

x_A = x_R - v_{x_R}t_R
y_A = y_R
z_A = z_R
t_A = t_R

and gives them to Observer_A to review. Both observers conclude that the coordinate transformation is valid when the subscript "R" stands for the "Observer at Rest", not restaurant (R), since each finds themselves at rest and the other is moving. This transformation is old news that stood for about 300 years.

Galilean Transformation

Galileo Galilei (1564 - 1642) treated time as an absolute and equated Reference Frames by four relationships now called the "Galilean Transformation". This was later refined by Hendrik Lorentz (1853 - 1928) due to experimental evidence that an Observer_A and an Observer_R may measure different distances, elapsed times, and even different orderings of events. The Galilean Transformation is a special case of the "Lorentz Transformation". The Principle of Relativity remains fundamental and valid.

What does Physics measure?

There is a large technical vocabulary used in Physics but a lot can be understood with only three words. In the four experiments

Dropping a coin,
Propelling an ice cube to a constant velocity,
Balancing two ice cubes, and
Colliding two ice cubes

in two Frames of Reference several Physical Properties can be determined. The Principle of Relativity is a fundamental truth (Small "t" truth) which can be taken for granted as long as it is not violated by an error in reasoning. Velocity is calculated from a measure of Length[L] and a measurement of Time[T].

[L]
-----
[T]

Velocity =

A measure of Length can be measured in shorter or longer distances but Length itself is what it is. A measure of Time can be measured in smaller or larger duration but Time itself is what it is. "so far so good"

In less than half-a-blink-of-an-eye, in the second experiment, the ice cube is moving at a constant velocity. It took a bit of Time for the ice cube to get up to speed. The result of this brief passage of Time is velocity. Something multiplied by Time resolves into velocity. This something is

[L]
---------
[T][T]

Acceleration =

While the spring was in contact with the ice cube and decompressed it accelerated the ice cube to a velocity which remained constant once the spring was no longer in contact. There is something in the compressed spring that is transferred to the stationary ice cube resulting in an uncompressed spring and a moving ice cube. There is also another something missing just about the ice cube. It the first state it is at rest. In its second state it is in motion. A closer look at history and the other experiments can reveal what this transfer is and what these two "somethings" are.

For countless ages it has been observed that it takes effort to start an object moving and it takes effort to stop its motion. To understand this need for effort the concept of Inertial Mass [M_I] was invented. A concept must be testable and provide valid predictions of events to be accepted in Physics.

The "effort" is provided by the spring where the "transfer" is expressed by force acting on the Inertial Mass of the ice cube. When the ice cube is in contact with the spring, the ice cube experiences and acceleration. Force can be defined in terms of Inertial Mass and acceleration.

[M_I][L]
---------
[T][T]

Force =

The theory states that the force accelerates the ice cube to a constant velocity which is verified experimentally. Since the spring provides an equal amount of force on an ice cube for each repetition of the experiment the theory predicts that changing [M_I] will change the velocity in a specific way.

[M_I][L]
---------
[T][T] [M_I][L]
---------
[T]

× [T] =

The resultant velocity is inversely proportional to the magnitude of the Inertial Mass. This is also confirmed in the experiment when it was observed that lighter ice cubes move faster than heavier ice cubes. This line of reasoning and collection of experimental evidence validates the existence of [M_I] at least qualitatively.

In the first experiment the coin starts at rest, acquires motion upon release then returns to rest on the table. Holding the coin suppresses its motion until it is released and then the table suppresses the motion again.

The scientific investigation into gravity dates back to at least the 4^th century BC. The physics of Aristotle (384 BC - 322 BC) is a philosophy broader in scope than Modern Physics. It set forth principles of change that govern the natural world and laid the foundation of scientific reasoning. Aristotle's concept of gravity was refined by Galileo (1564 - 1642), Isaac Newton (1642–1727), and physicists to the present day.

This experiment demonstrates gravity acting on the coin. When released the coin accelerate into a state of motion until it comes to rest again on the table. Centuries of experimentation have determined that all bodies accelerate at the same rate near the surface of the Earth. Yet all bodies do not weigh the same. Gravity is acting on some property of the body. That something about matter is named Gravitational Mass [M_G]. Acceleration due to gravity is a property in Space surrounding a body of matter. The acceleration produces motion unless it is restrained by something equal and opposite to the direction of the acceleration. The magnitude and direction is called the

Normal Force = - (Gravitational Mass) × (acceleration due to gravity)

thus what we experience as weight is the retarding effect the normal force on the motion of an of a body acted on by the acceleration due to gravity. Without a normal force between the table and the coin, the coin would move though the table at an ever increasing velocity. The concept of Gravitational Mass [M_G] is consistent with the principles and calculations based on experimental measurements thus far and it offers a testable explanation of weight. "so far so good" Rearranging the equation for the Normal Force

[M_G][L]
---------
[T][T]

- Normal Force = Weight = Force =

reveals an equation in the same form which was derived to describe the properties of Inertial Mass [M_I].

This formalism explains some observed phenomena. The force needed to hold the coin above the table is the same amount of force the table exerts on the coin when it is lying motionless. This is commonly called weight and specifically called the weight of the coin in this experiment. It appears the acceleration due to gravity acts on [M_G] when objects are in contact and at a distance from each other.

The conclusion that the force needed to hold an ice cube motionless in contact with a compressed spring is derived from the measurement of its velocity when it is released. The acceleration experienced by [M_I] exists during the brief time of contact with the spring after the effort to hold the ice cube stationary is removed explains why the ice cube moves with a constant velocity. Acceleration stopped when contact stopped.

A strange thought just occurred. Is a Falling Object Weightless? There is no Normal Force acting on the object to give a perception of Weight.

A conclusion can be made from the results of the third experiment that when two ice cubes are in balance on equidistant points from the center of the rule they experience the same amount of Normal force to arrest the motion that the acceleration due to gravity would impart. Their weights are equal and their [M_G] are equal. Using the ice cubes from the third experiment in the forth experiment allows the comparison of [M_G] to [M_I]. After the collision of ice cubes the final velocity of two ice cubes traveling in contact together was found to be half of the initial velocity of the single ice cube traveling alone. When two ice cubes were already in contact and accelerated into motion by the spring their velocity was equal to the final velocity of the collision experiment. Inertial Mass [M_I] and Gravitational Mass [M_G] appear to be proportional based on the experiments and the theoretical construction of Physics derived thus far.

Centuries of experimentation verify that [M_G] = [M_I] to an accuracy of about twenty decimal places. That is [M_G] = [M_I] to one part in a hundred thousand thousand thousand billion which is one part over twenty orders of magnitude which is a ratio of 1 : 10²⁰ which is rather unimaginable. OK, a billion is 10⁹ and light travels about 1 foot in a billionth of a second. A billionth of a second is called a nanosecond, 10^-9 second. Global Positioning Satellites (GPS) measure time in nanoseconds to calculate positions on Earth. Modern Physics Equations models this stuff and interactions, but for now... Mass [M] is Mass[M]. It interacts with farces and gravity.

Length[L], Time[T], and Mass[M] are Fundamental for now

The square bracket notation is used to designate dimension with reference to a coordinate system. Length can be measured in feet, meters, or whatever is convenient. Time can be measured in seconds, years, or whatever is convenient. Mass can be measured in slugs, grams, or whatever is convenient. A lot of Physics can be understood by comprehending the relationship of [L], [T], and [M].

Perception and Prediction: Part I

The Observer affects the Observed is consistent with the Principle of Relativity and taken together form a Philosophical Basis of Physics. The truths of Physics are validated by experimental evidence and may well point to the Truth of what the Universe is and how it works. What follows in these sections "Perception and Prediction: Part ..." are interpretations of observations consistent with Physics Equations.

It takes more effort to lift a ball from the ground to a waist high position than to simply support it waist high above the ground. It takes some skill to catch a thrown ball. Sometimes it will sting your hand upon impact but other times it seems to gently come to rest. It both cases after a successful catch you support the weight of the hall in your hand. The weight of the ball remains constant during the interactions yet the ball "feels" different at times. The weight is the force due to the acceleration due to gravity directed towards the ground interacting with the mass of the ball

[M][L]
---------
[T][T]

F = ma =

for short. The "F" and "a" are in bold type to represent vector quantities, having both magnitude and direction. The ball has a different feel when you change its velocity. At the moment you pick the ball up it feels slightly heavier, then "normal", then slightly lighter for an instant when you stop its motion at your waist. You accelerated it into motion, "heavier", and decelerated it to a stop, "lighter", while most of the time it felt normal. The sensation of heavier and lighters more dramatic if you have the opportunity to take a freight elevator from say the Loading Dock up to the third or forth floor.You will feel heavier when it starts and lighter when it stops while feeling motionless when traveling upwards at a uniform speed.When you catch a ball by stopping it quickly it can sting your hand. If you cradle the ball during the catch it stops more slowly and you do not hurt your hand.

We seem to have an intuitive perception of acceleration, velocity, and force. We also have a lot of words to describe these three thing, so many in fact that it would make Physics sloppy. Acceleration and decelerated are simply referred to as acceleration. Velocity is a vector which has a magnitude called speed. Say a race car is traveling on a mile long circular track. It completes a lap every 18 seconds, so it has an average and instantaneous speed of 200 miles per hour because speed has no directional component. Its average velocity per lap is zero because the directional component rotates 360° with each lap and sums to zero per lap. Note of caution: The instantaneous velocity at every point on the car's path of 200 miles per hour. Check both vectors before crossing the track.

The way the ball "feels" is related to it mass and velocity which is called momentum, specifically linear momentum

[M][L]
-------
[T]

p = mv =

and the change of momentum is felt as a force. Note that "lifting" the ball from the floor and "impact" of the ball when catching it are two different words but in physics are the same word, "force".

Newton's Laws of Motion are mathematical expressions of perception experimentally verified by Physics. By the IOTTMCO Theorem

The IOTTMCO Theorem ≡ Intuitively obvious to the most casual observer

Newton's First Law: Every object will remain at rest or in uniform motion in a straight line unless compelled to change its state by the action of an external force.

Newton's Second Law: The rate of change of momentum, acceleration, produced by a particular force acting on a body is directly proportional to the magnitude of the force, inversely proportional to the mass of the body and in the same direction of the particular force.

Newton's Third Law: To every action there is always opposed an equal reaction; or, the mutual actions of two bodies upon each other are always equal, and directed to contrary parts. "For every action there is an equal and opposite reaction."

but, it is not warranted to use such a Powerful Mathematical Theorem.

A Closer Look at Newton's Laws of Motion

The support for these three laws can be found in the preceding words of this paper which draws from multiple sources including

Philosophiæ Naturalis Principia Mathematica, Latin for "Mathematical Principles of Natural Philosophy", often referred to as simply the Principia, is a work in three books by Sir Isaac Newton, first published 5 July 1687. After annotating and correcting his personal copy of the first edition, Newton also published two further editions, in 1713 and 1726. The Principia states Newton's laws of motion, forming the foundation of classical mechanics, also Newton's law of universal gravitation, and a derivation of Kepler's laws of planetary motion (which Kepler first obtained empirically). The Principia is "justly regarded as one of the most important works in the history of science".

http://en.wikipedia.org/wiki/Philosophi%C3%A6_Naturalis_Principia_Mathematica

such that

Newton's First Law: p = mv

Newton's Second Law: F = ma

Newton's Third Law: F₁₂ = - F₂₁

is comprehensible within the scope of this paper.

This Should Really Be A Footnote: E1

What is work? Something you do to make money. Something you do to make a change. The active effort to stop a change from happening. I worked as a Nurse for more than two decades, it did not seem like work most days because I enjoyed it. Perception along with the observed effecting the observer slips into the definition of work. Work is the observed effect of effort. Among the many definitions of work there are consistencies that "work" in Physics. The effort of the spring makes a change on the ice cube. The work, W, done by the spring is the result of the force, F, effecting the ice cube during the contact distance, d, producing motion in the direction parallel to F and d. Work does not have a direction. It is a positive magnitude indicative of what is interesting to the observer.

W = Fd

the dimensions of mechanical work

[M][L][L]
-----------
[T][T]

W = Fd =

and the scalar product of two vectors is governed by mathematical reasoning

w = F⋅d ≡ |F| |d| cos θ = F_xd_x + F_yd_y + F_zd_z

the Dot Product.

The choice of coordinates is a matter of convenience made by the observer. The use of square bracket has introduced the field of Dimensional Analysis. This field will be explored further as needs in Physics arise.

This Should Really Be A Footnote: E2

What is energy? The ability to do work. The follow up question, "But, what is it?" usually elicits a rather long list including: acoustic, chemical, elastic, electric, gravitational, luminous, magnetic, mechanical, nuclear, optical, radiant, solar, sound, thermal, vibrational, and mass itself which are all forms of energy. They come with their own units of measurement, some of the words in the list have overlapping meanings and some can be divided into sub categories. Through this confusion of observation and nomenclature it can be stated that energy is indeed one "thing" and the cacophony of units can be stated in one relationship.

Newton's First Law: p = mv is validated by observation and reason. What unobserved quantity maintains the objects momentum, p, and velocity, v, while being consistent with the Physics developed thus far? This unobserved quantity is a property of the object "maintaining" the velocity of the object. This is a very simplistic interpretation of the unobserved quantity, energy, and has limited usefulness. Energy, E, is hypothesised to be a scalar with the following dimensions

[M][L][L]
-----------
[T][T]

E = pv = mvv =

When the moving ice cube collided with the stationary cube it did work as measured by the change in motion of the stationary ice cube. Interestingly, the total momentum before and after a collision does not change. It is distributed between the objects. Momentum is said to be conserved property within a system that does not experience external forces. This turn out to be true for any number of colliding objects. The same concepts of conservation, interactions by forces, work, distribution of motions, and maintaining of the velocity of objects is shared by the unobserved quantity defined as energy.

Defining energy with the dimensions [M][L]²/[T]² based on an intuitive philosophical leap or an IOTTMCO guess based on algebraic manipulation and observation turned out to be a "really good guess". The most recognized equation in the World

E = mc²

has the same dimensions. It is credited to Albert Einstein from his 1905 paper "Does the Inertia of a Body Depend upon its Energy-Content?" which surprisingly does not has the equation written in its famous form.

E
---
c² hν
---
c² mc²
----
h

m = = <=> ν = <=> E = mc²

The important point in this Footnote is that "Energy is Energy. All the names and units used to measure it are really the same. Energy can be neither created nor destroyed, only change from one form to another". As for Einstein's Equation with Plank's Constant, h, frequency, ν, and speed of light, c, keeping mass, m, and energy, E, in proportion will require a bit more Physics to grasp.

A Comment On Refinement

Billy Pilgrim is a character in the book, "Slaughterhouse-Five" written by Kurt Vonnegut Jr., who has come unstuck in time. Comprehending Physics Equations share this quality with Billy Pilgrim. If you find a book or three by Kurt Vonnegut Jr. you will find them multi-facetted, woven with rich textured layers of storylines and plot making them all thought provoking and entertaining.

The understanding of the construction of a universe can be told in a historical chronology of discovery or built from fundamentals. However, certain fundamentals require a historical context to be made understandable. Physics is and always has been a developing science of discovery and refinement. A telling of this tale combining the chronology and the build feels reasonable. The six basis elements: existence, observation, length, time, relativity, and mass have yielded explanations for and relationships among a handful of physical phenomena. Everything stated in this paper so far is valid and consistent, but some of these thing will be found to be special cases of a more general or refined principle. It all still works and it works more completely when more is observed and understood. The need for further refinement will be addressed in the following pages to the extent that I am able. Further refinement where needed will be through the efforts of the reader. To this end I say "Thank you".

IOTTMCO Discoveries Explained

Everything is in motions. When I say something is at rest, not in motion, it underscores the bias I have that it is not moving relative to me. After all, I am the center of the Universe. This bias is acceptable because anyone can say it and determine the fundamental Laws of the Universe and be in agreement with everyone else. Newton's Three laws of Motion

Newton's First Law: p = mv
Every object will remain at rest or in uniform motion in a straight line unless compelled to change its state by the action of an external force.
Newton's Second Law: F = ma
The rate of change of momentum, acceleration, produced by a particular force acting on a body is directly proportional to the magnitude of the force, inversely proportional to the mass of the body and in the same direction of the particular force.
Newton's Third Law: F₁₂ = - F₂₁
To every action there is always opposed an equal reaction; or, the mutual actions of two bodies upon each other are always equal, and directed to contrary parts. "For every action there is an equal and opposite reaction."

communicate the the base principles between moving frames. Using algebra and calculus to combine these Three Laws render a large number of models for the Physical World. Many phenomena look and feel different but they are essential the same.

Acceleration due to Gravity, Weight, Normal Force, and Friction

When you stand on the floor you feel the Force of Gravity pulling you down. Actually, there is no "Force of Gravity". There is an Acceleration due to gravity that is retarded by the Normal Force of the object that is supporting you, but it is convenient and practical to calculate the acceleration as a force. Say, a friend drops a baseball out of a second story window. You catch it, momentarily retarding its acceleration, and then drop it. No big deal. But say your friend, drops a grand piano out the window. You do not want to repeat the experiment even though the ball and piano would have the same acceleration at the height of your hand.

Or consider, you are walking up a steep hill of about 30 degrees. You feel a resistance to each step taken which is not felt when walking on a level surface. If you slip, you accelerate downhill parallel to the grade. The perception of a force parallel to the slant of the hill and opposite to the direction you are walking is undeniable; but what is generating this force? Gravity is accelerating you straight down, vertical, and the hill is exerting a normal force to support you. The Normal Force is perpendicular to the surface of the hill and is 30 degrees less than vertical. The magnitude of the Normal Force is less than your weight, N = mg cos θ, proportional to the angle of elevation. Adding your Weight (force) and the Supporting force (Normal Force) reveals the small perceived force tugging you downhill. When you are able to stand motionless on the incline it is because the tugging force is balanced by an equal and opposite Frictional Force. A frictional force is proportional to the Normal Force, the substances in contact and their relative motion perpendicular to the Normal Force.

Static Frictional Force: F_s ≤ μ_sN
Kinetic Frictional Force: F_k = μ_kN

Newtonian Mechanics provides a framework for describing the Universe defined by the Kinematics and Dynamics of a System. Where the System is a neighbourhood of the Universe that can be measured. The measurements may be made directly or indirectly depending on its scale relative to Humans and Technology. Kinematics is a complete description of the Present: Length[L], Time[T], and Mass[M] are Fundamental for now. Dynamics is the cause of change from past to present to future.

A wise man once said, "Think about Physics with a pencil". To this I would add, "Expand and contract the drawings and equations to capture the Detail and Fundamental".

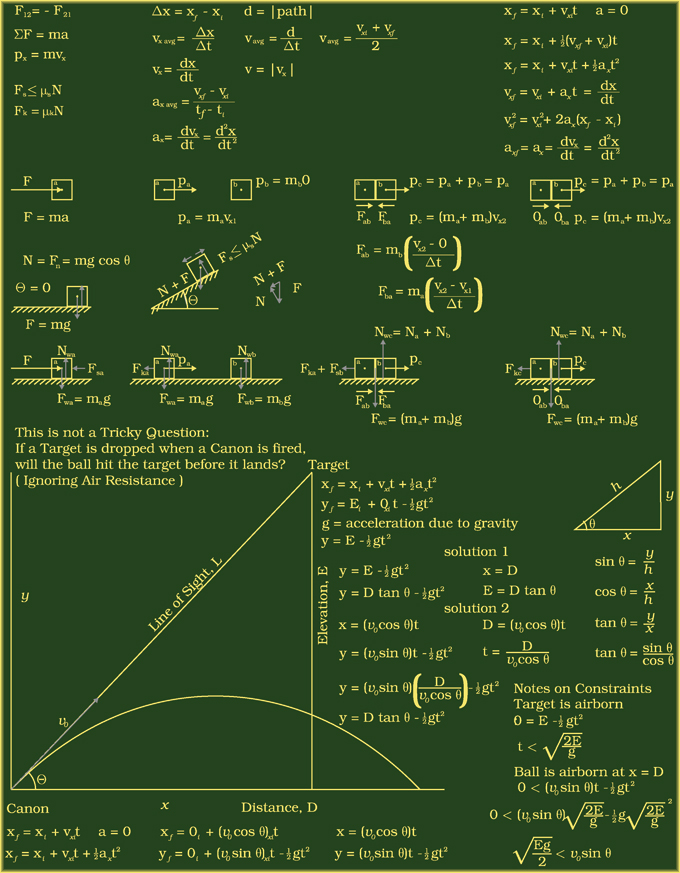
This is not a Tricky Question: Throwing Stones

What goes up must come down is true, at least in a Gravitational Field. The diagram above is billed in Circuses and Physics Courses as "The Perfect Shot". If a Canon is aimed directly at a Target that is at a distance D and at height E is fired when the Target is dropped, the Ball will always hit Target before it impacts the Ground when the Ball exists the Canon with enough speed.
There are two models in this system:

The falling Target

x = D

1
---
2

y = E - g t²

The Projectile

x = (v₀cosθ)t

1
---
2

y = (v₀sinθ)t - g t²

and "The Perfect Shot" have identical (x, y, t) coordinates when the Ball hits the Target. Heeding the words of the wise man, "Think about Physics with a pencil" simplifies solving four simultaneous equations.

Since I am not to thrilled about solving simultaneous equations and somewhat suspicious of Trigonometry with its "Where the heck did that come from" Identities. Lets look at what Newton's Models are actually saying.

The falling Target x = D
A. there is no horizontal acceleration
B. there is no horizontal velocity
The falling Target y = E - ½gt²
A. there is vertical acceleration
B. there is no initial vertical velocity
C. the Target starts moving at a height of E
D. The target hit the Ground at time, t = √2E/g
The Projectile x = (v₀cosθ)t
A. there is no horizontal acceleration
B. there is an initial horizontal velocity, v₀cosθ which remains constant
C. the Ball must be at x = D when time, t < √2E/g
The Projectile y = (v₀sinθ)t - ½gt²
A. there is vertical acceleration
B. there is an initial vertical velocity, v₀sinθ which means the Ball is airborne for y > 0
C. the Ball must be at y = (v₀sinθ)t - ½gt² when the Target is at y = E - ½gt²

So, with a little Algebra I can find the exact (x, y, t) coordinates, but if i just want to set up the Circus Act so it works every time all I need is 3.C and 4.c

t < √2E/g
(v₀sinθ)t - ½gt² = E - ½gt²

(v₀sinθ)t = E

be careful with inequalities

(v₀sinθ)(√2E/g) < E

- say what you mean and mean what you say

v₀sinθ > √Eg/2

The truth be told. This is the forth time I have thought about this "The Perfect Shot" system. The Zeroth Step was drawing the picture and writing down Newton's equations. Solution2 came next with just using trigonometry and Algebra. Solution1 came next by looking at the picture and "seeing" similar triangles. The "Notes on Constraints" dawned on me when I realized solutions 1 and 2 where a lot of work that did not answer my question. The final solution, written on this page was an effort to Reduce the System to its simplest parts. Saw that it rendered more information than I needed, albeit I am now completely confident that v₀sinθ > √Eg/2 will keep me indispensable to the Circus.

Motion and Gravity

Everything is in motion. Planets move around suns. Solar Systems orbit their neighbours, which in turn orbit the Galaxy. Clusters of galaxy dance their waltz through light years of distance. The web of galactic clusters hurtle through the Universe as far as the eye can see. Going small, the surface of the Earth is traveling about a thousand miles per hour around its core. We see objects moving all about us daily. We know about motions we cannot see and perceive them as pressure and temperature. By the "Principle of Relativity" we can pick a point and call it Zero. The chair next to the table are at rest. They have zero motion relative to each other. Many motions fade into the background and can be ignored, however Fundamentally the vast array of motions are present in the description of the System. Details ignored in the past become significant in the future when they are understood for their influence in the present.

Motion is inherent in our world. Newton's Laws, specifically this First Law: p = mv, demands a rewrite of the first sentence of this paragraph. Motion is inherited in our world. The best fit theory of the origin of motion is the "big Bang". The transfer of motions from "stuff" to "stuff" traces back to when only Energy existed and prior to the creation of stuff.

Newton's Law of Gravity has stood the test of time for over 300 years.

m₁m₂
------
r² [L][L][L]
-----------
[M][T][T]

F = G G = G = 6.67 × 10^-11 m³kg^-1s^-2

This law provides a description of how Gravity works and has always been silent as to what Gravity is. Objects move on an inert, unchanging stage where Time "ticks" uniformly from past to future and "now" is the same everywhere. Length and Time are two separate Fundamental entities in Newton's description which are immutable everywhere and every when.

The Theory of Gravity was refined in the early 20^th century by Albert Einstein to account for the motion of a relatively small mass object very close to a very large mass object. The implication was that the stage of Length and Time was not inert nor were Length and Time two separate Fundamental entities.

Einstein's Field Equations are a Piece of Cake

He developed a Notation System where one letter can stand for a set of equations. The set of equations are then consistent with the Operations defined by an equation of larger scope. Each term is more than arbitrary variables like y = f(x), though y is constrained by the value of x and x itself is constrained by the operation of f(). These steps of Constraints form a path to Concepts. Now step along a path of Concepts guided by the considerations laid down thus far, existence, observation, length, time, relativity, and mass.

Einstein's Field Equations Solutions come in various forms based on the level of detail desired and the application. Here is a form that serves as a basic comparison to Newton's Law of Gravity which is a specific case of Einstein's Field Equations for General Relativity

8πG
----
c⁴

G = T

where G measures curvature, G is the Newtonian gravitational Constant, c is the speed of light, and T measures matter content of the system.

The terms G and T are sets of equations imbedded in G = (8πG/c⁴)T which if expanded would obscure the concepts of curvature and matter content. "Matter tells Spacetime how to curve, and Spacetime tells matter how to move", said John Wheeler because he saw the link between G and T and knew what G and T are.

We will start with G and build Newton's stage out of Wooden Blocks, rows and columns to make a layer and then layer upon layer to fill a space. Every rigid block has its identical rigid neighbours. The edges of the blocks make up a rigid coordinate system identifying up-down, right-left, and forward-backward. No matter where you are Time "ticks" simultaneously and uniformly from past to future. If you are here and someone is over there, then something happens elsewhere. Everyone in Newtonian Space and Time agree when and where "it" happens. Observations revealed details that show Newton's Law of Gravity is a special case solution of the large scope description of gravity encapsulated by Einstein's Field Equations.

Replace the Wooden Blocks by soft stretchy spongy cubes. Each cube continues to "tick" Time from past to future. Each tick is the amount of Time it takes for light to traverse an edge from corner to corner. This "time scale" applied to Newton's Blocks by Einstein comes from Maxwell's Equations. There is much to be said about Maxwell's Equations, but for now all we need is the maximum "Speed of Light", c. The block can now be squished, stretched, twisted, dimpled, bumped, curved, concaved, convexed, and even flattened to resemble rigid Wooden Blocks. When a volume is compressed the lines of spacetime (space and time) get closer to each other, When stretched they diverge to increase the distance between events in space and time (spacetime).

In our everyday world we experience the lines of spacetime as parallel and evenly spaced. We each live on our own line of spacetime. If I agree to met you at Joe's Market a 3 o'clock and we both arrive on time our spacetime lines have converged. After picking up our Lottery Winnings we leave to go our separate ways, our spacetime lines have diverged. The distance of our spacetime paths we traveled to and from Joe's Market are determined by the soft stretchy spongy cubes of spacetime that envelope our Neighbourhood. The spacetime cubes are so close to the Newtonian Wooden Blocks that we get the same results we calculating the distances traveled and the time spent to pick up our Lottery Winnings. Truth be told, Newton's Wooden Blocks work for describing the orbit of the Moon and the Planets around the Sun. Except for a small detail about Mercury's Orbit.

The Wooden Blocks of Newton were somewhat squish and twisted. What's more, time played into the proper squish and twist of the Blocks so they could be dynamic enough to work at Joe's Market, the Moon and the orbit of the other Planets. The un-parallel-ness and un-evenly spaced lines of spacetime in the Neighbourhood of the Sun cause Mercury to fall in the observed orbit it has around the Sun.

So, "Spacetime tells matter how to move" is the G part of Einstein's Field Equation. "Lets have some Cake".

You come home one day and smell someone has been baking. A 9 by 13 inch White Cake is near. The aroma is intoxicating. Upon entering the kitchen a golden cake with just the hint of chocolate swirls on top meets your gaze. "No frosting, the deliciousness is in the Details", the Master-Baker slices a piece of Cake. A dense Brownie Mix had been swirled in to the lighter White Cake Batter. You can not fathom how they could have baked together. But, I digress. The textures delight your Taste. There is something else, robust and tantalisingly gooey. Yes, it's peanut butter but there is more. Small, sharp, hard, well defined. "My God, its full of heath toffee."

And that's what matter content T is, the ingredients keeping their distance one from another by their nature (mass and a few more) yet influencing the baking in the distance between (spacetime).

Paths not Taken

Here are things which fascinate me and slip from my grasp. My understanding of them is not deep nor wide enough to explain them to myself or in explaining one I lose my understanding of the world. These are the concepts in my realm of contemplation. I accept their shadows as guideposts to Understanding. The shadows are tools I humbly use to explain what I can, yet I am justified in do this by a Powerful Mathematical Theorem

The IOTTMCO Theorem ≡ Intuitively obvious to the most casual observer

The Mathematical Reasoning used to define the cosine function results in the Taylor serie

x²
---
2! x⁴
---
4! x⁶
---
6!

cos x = 1 - + - + ⋅⋅⋅

_∞
Σ
^{n = 0} (-1)ⁿx²ⁿ
----------
(2n)!

cos x =

The Mathematical Reasoning used to define the sine function results in the Taylor serie

x³
---
3! x⁵
---
5! x⁷
---
7!

sin x = x - + - + ⋅⋅⋅

_∞
Σ
^{n = 0} (-1)ⁿx^{2n + 1}
----------
(2n + 1)!

sin x =

Linear Algebra provides many mathematical tools used in Physics.

The number π is a Fundamental Relationship.